How to Develop a Mindset for Math

Math uses made-up rules to create models and relationships. When learning, I ask:

What relationship does this model represent?
What real-world items share this relationship?
Does that relationship make sense to me?

They're simple questions, but they help me understand new topics. If you liked my math posts, this article covers my approach to this oft-maligned subject. Many people have left insightful comments about their struggles with math and resources that helped them.

Math Education

Textbooks rarely focus on understanding; it's mostly solving problems with "plug and chug" formulas. It saddens me that beautiful ideas get such a rote treatment:

The Pythagorean Theorem is not just about triangles. It is about the relationship between similar shapes, the distance between any set of numbers, and much more.
e is not just a number. It is about the fundamental relationships between all growth rates.
The natural log is not just an inverse function. It is about the amount of time things need to grow.

Elegant, "a ha!" insights should be our focus, but we leave that for students to randomly stumble upon themselves. I hit an "a ha" moment after a hellish cram session in college; since then, I've wanted to find and share those epiphanies to spare others the same pain.

But it works both ways -- I want you to share insights with me, too. There's more understanding, less pain, and everyone wins.

Math Evolves Over Time

I consider math as a way of thinking, and it's important to see how that thinking developed rather than only showing the result. Let's try an example.

Imagine you're a caveman doing math. One of the first problems will be how to count things. Several systems have developed over time:

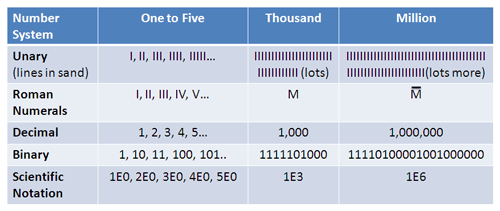
No system is right, and each has advantages:

Unary system: Draw lines in the sand -- as simple as it gets. Great for keeping score in games; you can add to a number without erasing and rewriting.
Roman Numerals: More advanced unary, with shortcuts for large numbers.
Decimals: Huge realization that numbers can use a "positional" system with place and zero.
Binary: Simplest positional system (two digits, on vs off) so it's great for mechanical devices.
Scientific Notation: Extremely compact, can easily gauge a number's size and precision (1E3 vs 1.000E3).

Think we're done? No way. In 1000 years we'll have a system that makes decimal numbers look as quaint as Roman Numerals ("By George, how did they manage with such clumsy tools?").

Negative Numbers Aren't That Real

Let's think about numbers a bit more. The example above shows our number system is one of many ways to solve the "counting" problem.

The Romans would consider zero and fractions strange, but it doesn't mean "nothingness" and "part to whole" aren't useful concepts. But see how each system incorporated new ideas.

Fractions (1/3), decimals (.234), and complex numbers (3 + 4i) are ways to express new relationships. They may not make sense right now, just like zero didn't "make sense" to the Romans. We need new real-world relationships (like debt) for them to click.

Even then, negative numbers may not exist in the way we think, as you convince me here:

You: Negative numbers are a great idea, but don't inherently exist. It's a label we apply to a concept.

Me: Sure they do.

You: Ok, show me -3 cows.

Me: Well, um... assume you're a farmer, and you lost 3 cows.

You: Ok, you have zero cows.

Me: No, I mean, you gave 3 cows to a friend.

You: Ok, he has 3 cows and you have zero.

Me: No, I mean, he's going to give them back someday. He owes you.

You: Ah. So the actual number I have (-3 or 0) depends on whether I think he'll pay me back. I didn't realize my opinion changed how counting worked. In my world, I had zero the whole time.

Me: Sigh. It's not like that. When he gives you the cows back, you go from -3 to 3.

You: Ok, so he returns 3 cows and we jump 6, from -3 to 3? Any other new arithmetic I should be aware of? What does sqrt(-17) cows look like?

Me: Get out.

Negative numbers can express a relationship:

Positive numbers represent a surplus of cows
Zero represents no cows
Negative numbers represent a deficit of cows that are assumed to be paid back

But the negative number "isn't really there" -- there's only the relationship they represent (a surplus/deficit of cows). We've created a "negative number" model to help with bookkeeping, even though you can't hold -3 cows in your hand. (I purposefully used a different interpretation of what "negative" means: it's a different counting system, just like Roman numerals and decimals are different counting systems.)

By the way, negative numbers weren't accepted by many people, including Western mathematicians, until the 1700s. The idea of a negative was considered "absurd". Negative numbers do seem strange unless you can see how they represent complex real-world relationships, like debt.

Why All the Philosophy?

I realized that my **mindset is key to learning. **It helped me arrive at deep insights, specifically:

Factual knowledge is not understanding. Knowing "hammers drive nails" is not the same as the insight that any hard object (a rock, a wrench) can drive a nail.
Keep an open mind. Develop your intuition by allowing yourself to be a beginner again.

A university professor went to visit a famous Zen master. While the master quietly served tea, the professor talked about Zen. The master poured the visitor's cup to the brim, and then kept pouring. The professor watched the overflowing cup until he could no longer restrain himself. "It's overfull! No more will go in!" the professor blurted. "You are like this cup," the master replied, "How can I show you Zen unless you first empty your cup."

Be creative. Look for strange relationships. Use diagrams. Use humor. Use analogies. Use mnemonics. Use anything that makes the ideas more vivid. Analogies aren't perfect but help when struggling with the general idea.
Realize you can learn. We expect kids to learn algebra, trigonometry and calculus that would astound the ancient Greeks. And we should: we're capable of learning so much, if explained correctly. Don't stop until it makes sense, or that mathematical gap will haunt you. Mental toughness is critical -- we often give up too easily.

So What's the Point?

I want to share what I've discovered, hoping it helps you learn math:

Math creates models that have certain relationships
We try to find real-world phenomena that have the same relationship
Our models are always improving. A new model may come along that better explains that relationship (roman numerals to decimal system).

Sure, some models appear to have no use: "What good are imaginary numbers?", many students ask. It's a valid question, with an intuitive answer.

The use of imaginary numbers is limited by our imagination and understanding -- just like negative numbers are "useless" unless you have the idea of debt, imaginary numbers can be confusing because we don't truly understand the relationship they represent.

Math provides models; understand their relationships and apply them to real-world objects.

Developing intuition makes learning fun -- even accounting isn't bad when you understand the problems it solves. I want to cover complex numbers, calculus and other elusive topics by focusing on relationships, not proofs and mechanics.

But this is my experience -- how do you learn best? A few friends have written up their experience: