1 Learning Strategy: Blurry To Sharp

What’s a better learning strategy: covering a subject in full detail from top-to-bottom, or progressively sharpening a quick overview?

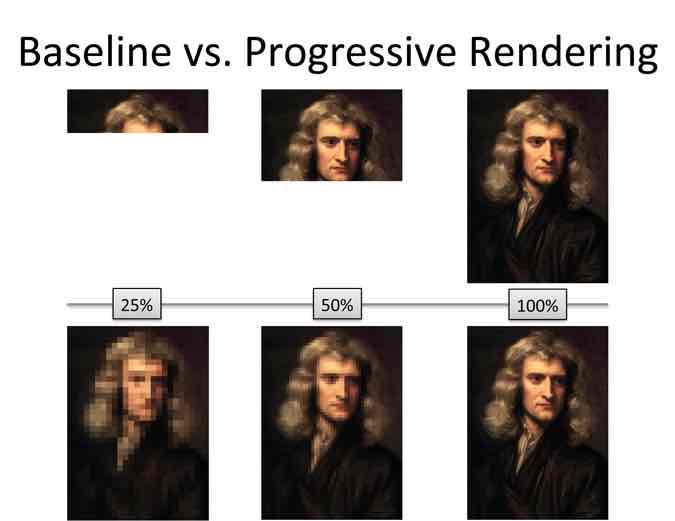
After a single class, which strategy gives you a better understanding of the material? Which helps you predict how later parts fit together? Which is more fun?

The linear, official, approach doesn’t work for me. Starting with a rough outline and gradually improving it keeps our interest and helps us see how the individual details are connected.

Most textbooks take the top-down approach, while this book is a blurry-to-sharp guide. The fine details are out there when you need them.

2 Learning Strategy: Appreciation To Performance

Next question: Do you need to be a musician to enjoy a song? No way.

There are several levels of music understanding:

• Intuitive Appreciation: Just enjoying the music.
• Natural Description: Humming a tune you heard or made up.
• Symbolic Description: Reading and writing the sheet music.
• Theory: Explaining how harmonies work, why minor scales are somber, etc.
• Performance: Playing the official instruments.

Math knowledge is the same. Start by appreciating, even enjoying, the idea. Describe it with your own words, in English. Then, learn the official symbols to make communication easier (“2 + 3 = 5” is better than “Two plus three equals five”, right?).

When we’re ready, we “perform” math by doing the calculations ourselves, and diving into the detailed theory. But only if you want! Decide for yourself what level of understanding you’d like to reach.

Here’s a guide to the book, depending on the level you’d like to reach:

• Intuitive Appreciation (Chapters 1-3)
• Technical Description (Chapters 4-5)
• Theory I (Chapters 6-8)
• Theory II (Chapters 9-14)
• Performance (Chapter 15)

The appendix includes a study plan if you wish to follow a formal course.

3 Learning Strategy: Study History

Calculus began when Archimedes realized shapes could be split into parts and rearranged. After a lifetime of effort, he discovered connections between spheres, circles and other shapes that were later etched onto his tomb.

Unfortunately, Calculus courses are taught out of order. The difficult, modern concepts are taught first (limits, developed 1800s) and the intuitive foundations are saved for the end (integrals, imagined 250 B.C.).

This book takes Archimedes’ approach: learn to see what Calculus can do, then layer in the theory as needed. By then, your interest has been piqued and you can read a modern textbook for the details (see recommendations in the final chapter).

Please don’t feel obligated to reach the Performance level of Calculus. For most of us (myself included), Appreciation and Description give practical, day-to-day insights. The first few chapters of this course are all that’s needed to have a better intuition than I had despite dozens of engineering classes.

Sound good? Let’s dive in.

4 Email Updates

BetterExplained.com provides high-quality, intuitive explanations to millions of readers each year. If you’d like updates on Calculus and related topics, sign up at:

http://betterexplained.com/newsletter

5 Book Webpage

Nobody likes typing URLs by hand. For clickable links for to the URLs in this book, notes, and other resources, visit:

http://betterexplained.com/calculus/book

6 The Legal Stuff

All content is copyright (c) 2015 Kalid Azad, except the following images used under Wikipedia’s Creative Commons license:

• “Portrait of Isaac Newton” by Sir Godfrey Kneller http://commons.wikimedia.org/wiki/File:GodfreyKneller-IsaacNewton-1689.jpg
• “Tree Rings” by Arnoldius http://commons.wikimedia.org/wiki/File:Tree_rings.jpg
• “Acropoclipse” by Elias Politis http://commons.wikimedia.org/wiki/File:Acropoclipse.jpg
• “Geometric Series” by Indolences http://commons.wikimedia.org/wiki/File:Geometric_series_14_square2.svg

You may use any of this material for educational use with attribution; for commercial use, please contact me using http://betterexplained.com/contact.