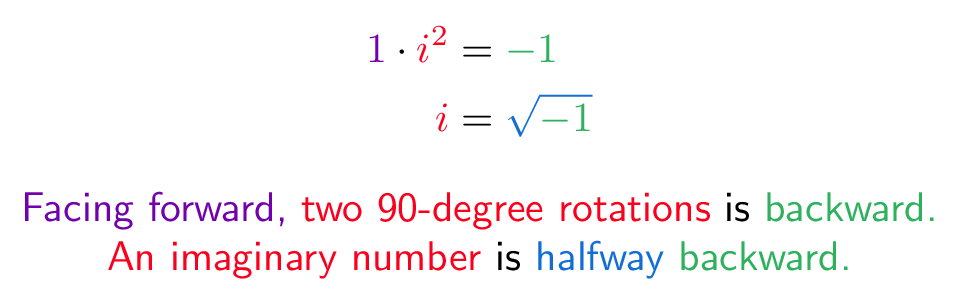A Visual, Intuitive Guide to Imaginary Numbers

Imaginary numbers always confused me. Like understanding e, most explanations fell into one of two categories:

• It’s a mathematical abstraction, and the equations work out. Deal with it.
• It’s used in advanced physics, trust us. Just wait until college.

Gee, what a great way to encourage math in kids! Today we’ll assault this topic with our favorite tools:

• Focusing on relationships, not mechanical formulas.
• Seeing complex numbers as an upgrade to our number system, just like zero, decimals and negatives were.
• Using visual diagrams, not just text, to understand the idea.

And our secret weapon: learning by analogy. We’ll approach imaginary numbers by observing its ancestor, the negatives. Here’s your guidebook:

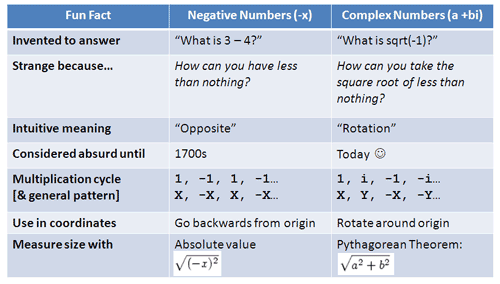
It doesn’t make sense yet, but hang in there. By the end we’ll hunt down i and put it in a headlock, instead of the reverse.

Video Walkthrough:

Really Understanding Negative Numbers

Negative numbers aren’t easy. Imagine you’re a European mathematician in the 1700s. You have 3 and 4, and know you can write 4 – 3 = 1. Simple.

But what about 3-4? What, exactly, does that mean? How can you take 4 cows from 3? How could you have less than nothing?

Negatives were considered absurd, something that “darkened the very whole doctrines of the equations” (Francis Maseres, 1759). Yet today, it’d be absurd to think negatives aren’t logical or useful. Try asking your teacher whether negatives corrupt the very foundations of math.

What happened? We invented a theoretical number that had useful properties. Negatives aren’t something we can touch or hold, but they describe certain relationships well (like debt). It was a useful fiction.

Rather than saying “I owe you 30” and reading words to see if I’m up or down, I can write “-30” and know it means I’m in the hole. If I earn money and pay my debts (-30 + 100 = 70), I can record the transaction easily. I have +70 afterwards, which means I’m in the clear.

The positive and negative signs automatically keep track of the direction — you don’t need a sentence to describe the impact of each transaction. Math became easier, more elegant. It didn’t matter if negatives were “tangible” — they had useful properties, and we used them until they became everyday items. Today you’d call someone obscene names if they didn’t “get” negatives.

But let’s not be smug about the struggle: negative numbers were a huge mental shift. Even Euler, the genius who discovered e and much more, didn’t understand negatives as we do today. They were considered “meaningless” results (he later made up for this in style).

It’s a testament to our mental potential that today’s children are expected to understand ideas that once confounded ancient mathematicians.

Enter Imaginary Numbers

Imaginary numbers have a similar story. We can solve equations like this all day long:

The answers are 3 and -3. But suppose some wiseguy puts in a teensy, tiny minus sign:

Uh oh. This question makes most people cringe the first time they see it. You want the square root of a number less than zero? That’s absurd! (Historically, there were real questions to answer, but I like to imagine a wiseguy.)

It seems crazy, just like negatives, zero, and irrationals (non-repeating numbers) must have seemed crazy at first. There’s no “real” meaning to this question, right?

Wrong. So-called “imaginary numbers” are as normal as every other number (or just as fake): they’re a tool to describe the world. In the same spirit of assuming -1, .3, and 0 “exist”, let’s assume some number i exists where:

That is, you multiply i by itself to get -1. What happens now?

Well, first we get a headache. But playing the “Let’s pretend i exists” game actually makes math easier and more elegant. New relationships emerge that we can describe with ease.

You may not believe in i, just like those fuddy old mathematicians didn’t believe in -1. New, brain-twisting concepts are hard and they don’t make sense immediately, even for Euler. But as the negatives showed us, strange concepts can still be useful.

I dislike the term “imaginary number” — it was considered an insult, a slur, designed to hurt i‘s feelings. The number i is just as normal as other numbers, but the name “imaginary” stuck so we’ll use it.

Visual Understanding of Negative and Complex Numbers

As we saw last time, the equation $x^2 = 9$ really means:

or

What transformation x, when applied twice, turns 1 to 9?

The two answers are “x = 3” and “x = -3”: That is, you can “scale by” 3 or “scale by 3 and flip” (flipping or taking the opposite is one interpretation of multiplying by a negative).

Now let’s think about $x^2 = -1$, which is really

What transformation x, when applied twice, turns 1 into -1? Hrm.

• We can’t multiply by a positive twice, because the result stays positive
• We can’t multiply by a negative twice, because the result will flip back to positive on the second multiplication

But what about… a rotation! It sounds crazy, but if we imagine x being a “rotation of 90 degrees”, then applying x twice will be a 180 degree rotation, or a flip from 1 to -1!

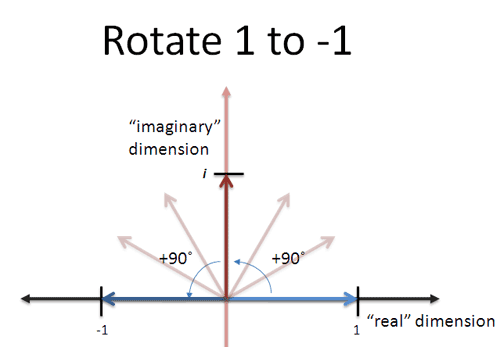
Yowza! And if we think about it more, we could rotate twice in the other direction (clockwise) to turn 1 into -1. This is “negative” rotation or a multiplication by -i:

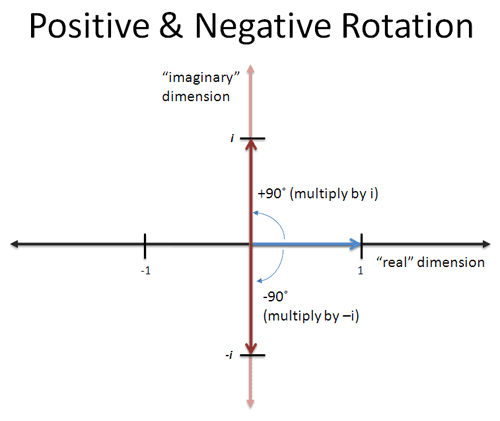
If we multiply by -i twice, the first multiplication would turn 1 into -i, and the second turns -i into -1. So there’s really two square roots of -1: i and -i.

This is pretty cool. We have some sort of answer, but what does it mean?

• i is a “new imaginary dimension” to measure a number
• i (or -i) is what numbers “become” when rotated
• Multiplying i is a rotation by 90 degrees counter-clockwise
• Multiplying by -i is a rotation of 90 degrees clockwise
• Two rotations in either direction is -1: it brings us back into the “regular” dimensions of positive and negative numbers.

Numbers are 2-dimensional. Yes, it’s mind bending, just like decimals or long division would be mind-bending to an ancient Roman. (What do you mean there’s a number between 1 and 2?). It’s a strange, new way to think about math.

We asked “How do we turn 1 into -1 in two steps?” and found an answer: rotate it 90 degrees. It’s a strange, new way to think about math. But it’s useful. (By the way, this geometric interpretation of complex numbers didn’t arrive until decades after i was discovered).

Also, keep in mind that having counter-clockwise be positive is a human convention — it easily could have been the other way.

Finding Patterns

Let’s dive into the details a bit. When multiplying negative numbers (like -1), you get a pattern:

• 1, -1, 1, -1, 1, -1, 1, -1

Since -1 doesn’t change the size of a number, just the sign, you flip back and forth. For some number “x”, you’d get:

• x, -x, x, -x, x, -x…

This idea is useful. The number “x” can represent a good or bad hair week. Suppose weeks alternate between good and bad; this is a good week; what will it be like in 47 weeks?

So -x means a bad hair week. Notice how negative numbers “keep track of the sign”: we can throw $(-1)^{47}$ into a calculator without having to count (”Week 1 is good, week 2 is bad… week 3 is good…“). Things that flip back and forth can be modeled well with negative numbers.

Ok. Now what happens if we keep multiplying by $i$?

Very funny. Let’s reduce this a bit:

• $1 = 1$ (No questions here)
• $i = i$ (Can’t do much)
• $i^2 = -1$ (That’s what i is all about)
• $i^3 = (i \cdot i) \cdot i = -1 \cdot i = -i$ (Ah, 3 rotations counter-clockwise = 1 rotation clockwise. Neat.)
• $i^4 = (i \cdot i) \cdot (i \cdot i) = -1 \cdot -1 = 1$ (4 rotations bring us “full circle”)
• $i^5 = i^4 \cdot i = 1 \cdot i = i$ (Here we go again…)

Represented visually:

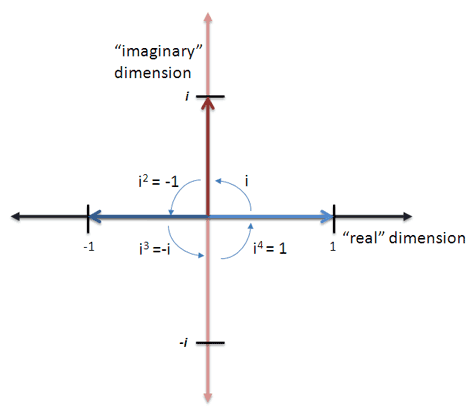
We cycle every 4th rotation. This makes sense, right? Any kid can tell you that 4 left turns is the same as no turns at all. Now rather than focusing on imaginary numbers ($i$, $i^2$), look at the general pattern:

• X, Y, -X, -Y, X, Y, -X, -Y…

Like negative numbers modeling flipping, imaginary numbers can model anything that rotates between two dimensions “X” and “Y”. Or anything with a cyclic, circular relationship — have anything in mind?

‘Cos it’d be a sin if you didn’t. There’ll de Moivre be more in future articles. [Editor’s note: Kalid is in electroshock therapy to treat his pun addiction.]

Understanding Complex Numbers

There’s another detail to cover: can a number be both “real” and “imaginary”?

You bet. Who says we have to rotate the entire 90 degrees? If we keep 1 foot in the “real” dimension and another in the imaginary one, it looks like this:

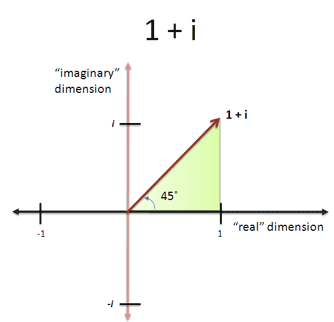
We’re at a 45 degree angle, with equal parts in the real and imaginary (1 + i). It’s like a hotdog with both mustard and ketchup — who says you need to choose?

In fact, we can pick any combination of real and imaginary numbers and make a triangle. The angle becomes the “angle of rotation”. A complex number is the fancy name for numbers with both real and imaginary parts. They’re written a + bi, where

• a is the real part
• b is the imaginary part

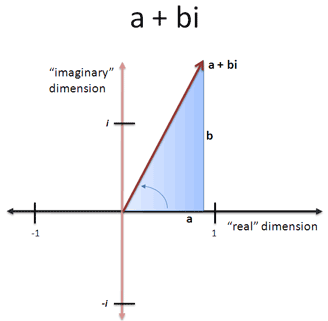
Not too bad. But there’s one last question: how “big” is a complex number? We can’t measure the real part or imaginary parts in isolation, because that would miss the big picture.

Let’s step back. The size of a negative number is not whether you can count it — it’s the distance from zero. In the case of negatives this is:

Which is another way to find the absolute value. But for complex numbers, how do we measure two components at 90 degree angles?

It’s a bird… it’s a plane… it’s Pythagoras!

Geez, his theorem shows up everywhere, even in numbers invented 2000 years after his time. Yes, we are making a triangle of sorts, and the hypotenuse is the distance from zero:

Neat. While measuring the size isn’t as easy as “dropping the negative sign”, complex numbers do have their uses. Let’s take a look.

A Real Example: Rotations

We’re not going to wait until college physics to use imaginary numbers. Let’s try them out today. There’s much more to say about complex multiplication, but keep this in mind:

• Multiplying by a complex number rotates by its angle

Let’s take a look. Suppose I’m on a boat, with a heading of 3 units East for every 4 units North. I want to change my heading 45 degrees counter-clockwise. What’s the new heading?

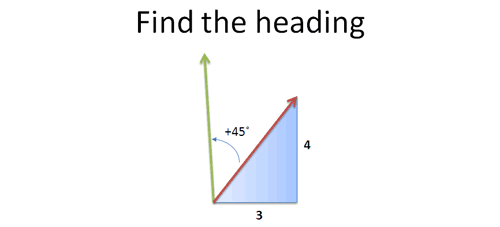
Some hotshot will say “That’s simple! Just take the sine, cosine, gobbledegook by the tangent… fluxsom the foobar… and…“. Crack. Sorry, did I break your calculator? Care to answer that question again?

Let’s try a simpler approach: we’re on a heading of 3 + 4i (whatever that angle is; we don’t really care), and want to rotate by 45 degrees. Well, 45 degrees is 1 + i (perfect diagonal), so we can multiply by that amount!

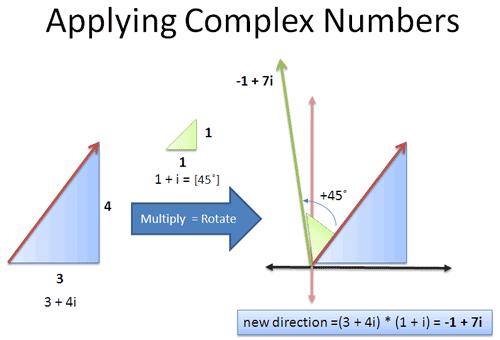
Here’s the idea:

• Original heading: 3 units East, 4 units North = 3 + 4i
• Rotate counter-clockwise by 45 degrees = multiply by 1 + i. (Here's why multiplication, not addition, performs the rotation.)

If we multiply them together we get:

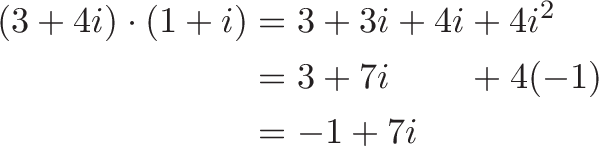
So our new orientation is 1 unit West (-1 East), and 7 units North, which you could draw out and follow.

But yowza! We found that out in 10 seconds, without touching sine or cosine. There were no vectors, matrices, or keeping track what quadrant we are in. It was just arithmetic with a touch of algebra to cross-multiply. Imaginary numbers have the rotation rules baked in: it just works.

Even better, the result is useful. We have a heading (-1, 7) instead of an angle (atan(7/-1) = 98.13, keeping in mind we’re in quadrant 2). How, exactly, were you planning on drawing and following that angle? With the protractor you keep around?

No, you’d convert it into cosine and sine (-.14 and .99), find a reasonable ratio between them (about 1 to 7), and sketch out the triangle. Complex numbers beat you to it, instantly, accurately, and without a calculator.

If you’re like me, you’ll find this use mind-blowing. And if you don’t, well, I’m afraid math doesn’t toot your horn. Sorry.

Trigonometry is great, but complex numbers can make ugly calculations simple (like calculating cosine(a+b) ). This is just a preview; later articles will give you the full meal.

Aside: Some people think “Hey, it’s not useful to have North/East headings instead of a degree angle to follow!”

Really? Ok, look at your right hand. What’s the angle from the bottom of your pinky to the top of your index finger? Good luck figuring that out on your own.

With a heading, you can at least say “Oh, it’s X inches across and Y inches up” and have some chance of working with that bearing.

Complex Numbers Aren’t

That was a whirlwind tour of my basic insights. Take a look at the first chart — it should make sense now.

There’s so much more to these beautiful, zany numbers, but my brain is tired. My goals were simple:

• Convince you that complex numbers were considered “crazy” but can be useful (just like negative numbers were)
• Show how complex numbers can make certain problems easier, like rotations

If I seem hot and bothered about this topic, there’s a reason. Imaginary numbers have been a bee in my bonnet for years — the lack of an intuitive insight frustrated me.

Now that I’ve finally had insights, I’m bursting to share them. But it frustrates me that you’re reading this on the blog of a wild-eyed lunatic, and not in a classroom. We suffocate our questions and “chug through” — because we don’t search for and share clean, intuitive insights. Egad.

But better to light a candle than curse the darkness: here’s my thoughts, and one of you will shine a spotlight. Thinking we’ve “figured out” a topic like numbers is what keeps us in Roman Numeral land.

There’s much more complex numbers: check out the details of complex arithmetic. Happy math.

Epilogue: But they’re still strange!

I know, they’re still strange to me too. I try to put myself in the mind of the first person to discover zero.

Zero is such a weird idea, having “something” represent “nothing”, and it eluded the Romans. Complex numbers are similar — it’s a new way of thinking. But both zero and complex numbers make math much easier. If we never adopted strange, new number systems, we’d still be counting on our fingers.

I repeat this analogy because it’s so easy to start thinking that complex numbers aren’t “normal”. Let’s keep our mind open: in the future they’ll chuckle that complex numbers were once distrusted, even until the 2000’s.

Carl Gauss, the famous mathematician, wrote:

"Hätte man +1, -1, √-1 nicht positiv, negative, imaginäre (oder gar ummögliche) Einheit, sondern etwa directe, inverse, laterale Einheit gennant, so hätte von einer solchen Dunklelheit kaum die Rede sein können."

"If +1, -1, √-1 had not been called a positive, negative, imaginary (or even impossible) unit, but rather a direct, inverse, lateral unit, then there could hardly have been any talk of such obscurity."

If you want more nitty-gritty, check out wikipedia, the Dr. Math discussion, or another argument on why imaginary numbers exist.

Other Posts In This Series

1. A Visual, Intuitive Guide to Imaginary Numbers
2. Intuitive Arithmetic With Complex Numbers
3. Understanding Why Complex Multiplication Works
4. Intuitive Guide to Angles, Degrees and Radians
5. Intuitive Understanding Of Euler's Formula
6. An Interactive Guide To The Fourier Transform
7. Intuitive Guide to Convolution
8. Intuitive Understanding of Sine Waves
9. An Intuitive Guide to Linear Algebra
10. A Programmer's Intuition for Matrix Multiplication
11. Imaginary Multiplication vs. Imaginary Exponents
12. Intuitive Guide to Hyperbolic Functions