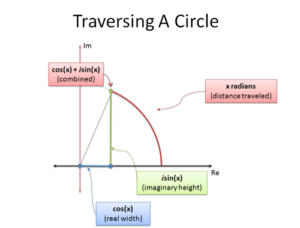
Euler's Identity
Colorized Definition
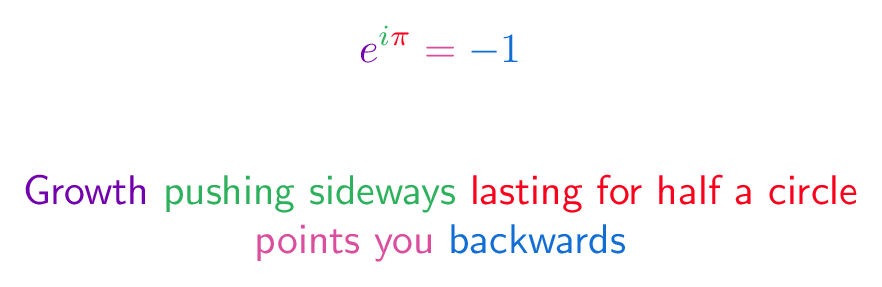
## Read More
- [Intuitive Understanding of Euler's Formula](https://betterexplained.com/articles/intuitive-understanding-of-eulers-formula/)
- [Math and Analogies](https://betterexplained.com/articles/math-and-analogies/)