You’re reading this, so I’ll assume your English is pretty good. What’s wrong with these phrases?
- Old little lady
- Red big dog
- Vietnamese spicy food
Do you have a logical reason for why they sound strange? Or are they just off?
You probably didn’t think, “In 3rd grade I mastered the Royal Order of Adjectives:
- Determiner
- Observation
- Size
- Shape
- Age
- Color
- Origin
- Material
- Qualifier
… and upon applying them, noticed several errors. Old little lady is incorrect because rules #3 and #5 are swapped — a childish mistake, really. The next…”
Ugh. Describing Gran Gran isn’t a logic puzzle. But guess what students learning English are taught?
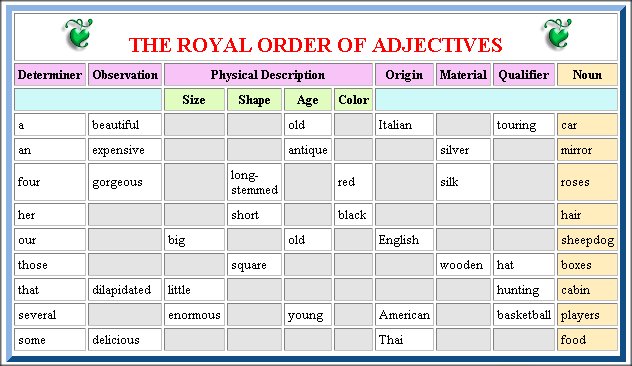
Even as a native speaker, could you construct this chart? Is this how you’d teach someone English?
The Adjective Fallacy is trying to learn by mastering the formal rules. Just because a concept can be rigorously defined doesn’t mean we should study it that way.
We didn’t become good at English by studying a chart: we developed an ear for the language and know how it should sound. And “old little lady” sounds off.
Similarly, getting good at math doesn’t mean marching through a gauntlet of rules on every problem. It’s having a native speaker’s feeling about what works or doesn’t.
“303 x 13 = 5074” looks strange, but not because we computed the left-hand side. It’s weird because odd numbers can’t multiply to become even (intuition). The last digit of the result should be 3×3= 9. 5074 is too large, since 300 x 10 (similar numbers nearby) is only 3000. Our Spidey Sense is blaring that the computation looks wrong.
My learning goal is knowing enough to make rough predictions on my own. I want a horse sense for algebra, calculus, trig, and even imaginary exponents, without scurrying off to apply an equation.
Rules aren’t inherently bad: they summarize, resolve ambiguous cases, and help us practice our weak spots. The question is how much to use them when starting off.
Learn enough rules to get started – don’t attempt to master them from the outset. See examples in a larger context and let the pattern-matching machinery of your brain get to work.
Learning Math
Math is a language too. Here’s a gut check: Would my current math study technique have helped me learn English?
If an English class spent a month on the adjective chart we’d have a talk with the teacher. But a Calculus class that spends weeks on the formal theory of limits is typical. Can we admit that studying this much detail, this early, doesn’t build fluency?
Pondering that question made me realize I had large gaps in trigonometry and calculus. I could only describe concepts using the adjective chart I’d memorized with a furrowed brow. (I’ll describe my grandma, just give me a minute!)
Enough was enough: embrace approaches that actually help you, like seeing the big picture first. In Calculus, that might mean seeing an integral in the first lesson:
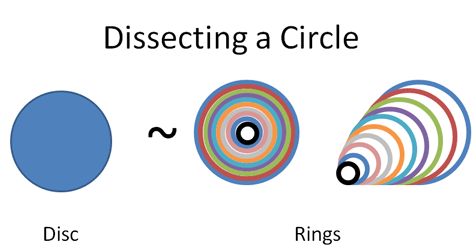
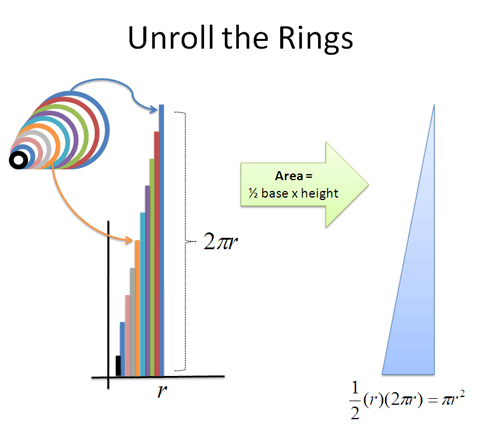
That’s what Calculus does: break a shape into pieces (the derivative), and glue it together in various ways (the integral). If you like this style of teaching, check out the full Calculus series.
A typical calculus syllabus covers integrals in week 12, after months of “building a foundation”. Better not use a complete sentence until we’ve studied adjectives, nouns and verbs separately, right? (My hand wringing could solve the energy crisis.)
The path to understanding isn’t always the most structured.
Happy math.
Update: This concept is called tacit knowledge, or “we know more than we can tell” (Michael Polanyi). Tacit knowledge is acquired through experience, and complements the explicit knowledge written as rules.
Other Posts In This Series
- Developing Your Intuition For Math
- Why Do We Learn Math?
- How to Develop a Mindset for Math
- Learning math? Think like a cartoonist.
- Math As Language: Understanding the Equals Sign
- Avoiding The Adjective Fallacy
- Finding Unity in the Math Wars
- Brevity Is Beautiful
- Learn Difficult Concepts with the ADEPT Method
- Intuition, Details and the Bow/Arrow Metaphor
- Learning To Learn: Intuition Isn't Optional
- Learning To Learn: Embrace Analogies
- Learning To Learn: Pencil, Then Ink
- Learning to Learn: Math Abstraction
- Learning Tip: Fix the Limiting Factor
- Honest and Realistic Guides for Learning
- Empathy-Driven Mathematics
- Studying a Course (Machine Learning) with the ADEPT Method




Leave a Reply
10 Comments on "Avoiding The Adjective Fallacy"
Your table has two errors: “old” and/or “Italian” do not go between “a” and “car.” I’m sure you know this. So I’m puzzled over why the errors? Is it to see how many people will comment, perhaps indicative of how many people actually read your blog? Regardless of the number, please keep up the good work. Your heart’s in the right place. Lord knows, we need help. I’m currently struggling with the meaning of discontinuities in functions of a complex variable. Can you help? Whether or not, thank you for your effort and kindness.
@George Frank:
I don’t see anything wrong with “a beautiful old Italian touring car”
If the square wooden hat boxes were old, would you say “square old wooden hat boxes”? I’d say “old square wooden hat boxes”.
Sorry, my mistake. I thought the words in the table were to be taken one at a time.
But I learned English when I was a baby, through constant immersion. I learned calculus in high school, an hour a day in a lecture. You can’t take just *some* aspects of one method, and plop them down in another context, and expect them to work. Adults don’t learn the same way babies do. You can’t pick up intuition in 5 hours a week the same way you can if you’re exposed 100 hours a week.
Once you know the basics, then you can learn the more subtle and complex rules through intuition and immersion. Once you know the meaning of all those adjectives, somebody can tell you “Old little lady sounds wrong. Say little old lady instead.” Even in (native) English class, we had to drill the basics by rote. We had weekly quizzes on vocabulary and spelling and so forth.
What you’re proposing sounds kind of like trying to teach the basics through intuition. That’s like taking somebody who doesn’t know any of the adjectives, and saying “Old little lady” (FROWN!), “Little old lady” (SMILE!). They don’t understand the concepts so trying to teach through immersion is essentially just requiring them to derive the rules themselves.
This is also what a lot of dance instruction gets wrong. You know how some people say “I just can’t dance”, while other people say “Anybody can dance!”? Most dance teachers I’ve had say things like “More graceful!” or “Take a chance!” or “Just feel the music!” but if they haven’t taught you the basic vocabulary first, these instructions are meaningless to you. (I’m not *trying* to be ungraceful here.) In fact, there are a few basic principles of body movement, for any given style of dance. Once you learn those you’ll look pretty good, and be able to start thinking about higher-level instructions. But if you don’t know them, then no amount of “More graceful!” will help.
Alex, you make a very valid point. “You can’t take just *some* aspect of one method, and plop them down in another context.” But there’s an intuitive part to most learning that we need to pay attention to. I am trying to learn French, and while the dictionary and the rules of grammar are very important, indispensable, actually, I found that immersing myself in both the written and spoken language, is what made me want to go on. When I read as much as I could, the rules came much more easily–some part of my brain had absorbed them.
I think you’re spot on for describing the pedagogical approach needed to help persons inclined towards the major learning categories (kinetic, visual, and audible), and how ineffective our generic pedagogy fails many people.
The Socratic method is ineffective at scale, but the resources for more individualized education hasn’t been a priority. It would be nice to have a more modern approach.
Adults can learn languages. There are many approaches to that, the most efficient seem to be those that rely on their intuition in their native tongue. Children learn differently, 100% intuitively.
Math is a language (J.W.Gibbs). Adults can learn math. There are many approaches to that. The most efficient, I believe, are those that rely on their intuition in their native tongue. Children learn differently, 100% intuitively.
In my experience, teaching a 7 y.o. trigonometry, probabilities, set theory, and logic (with formulas and all) was easier than teaching teenagers. It’s different with solving problem that requires attention span and other abilities that develop later, but the basic understanding was there. In my own studies, intuition was always first and then I refined my understanding by more formal approach.
Funny, I remember asking myself as a kid, “Why does ‘Teenage Mutant Ninja Turtle’ sound weird in any other word order?” After decades of schooling, this is the first I’ve heard of the royal order of adjectives. Sadly, nearly all subjects, including foreign language, are taught in this backwards manner.
I love your work, Kalid!
@Benjamin: Good point. I think technology can help scale the Socratic method up a bit (people helping each other, teachers helping many students, sharing knowledge of what works.) I hope we can move more resources towards this.
@Valery: Thanks for the comment. I agree — with children, we seem to focus on historical techniques that actually work (immersion, intuition-first, examples, etc.), vs. the over-formalization that we *think* works for adults.
@Lance: Great example! I never thought about TMNT but wow, even that complex scenario can be understood tacitly. Glad you’re enjoying the site :).