3. Expanding Our Intuition

Hope you thought about the question from last time: how do we take our X-Ray strategies into the 3rd dimension?

Here's my take:

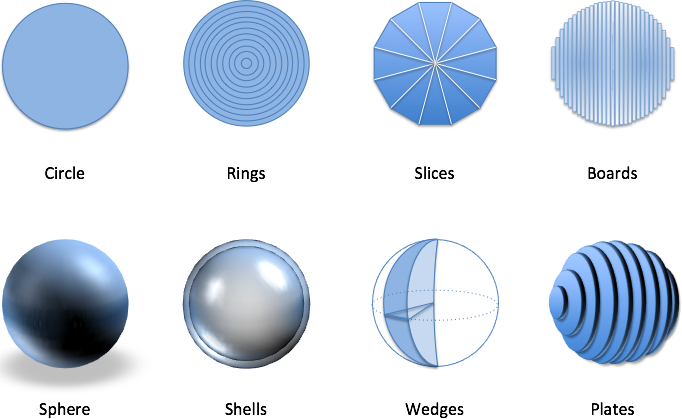
• Rings become shells, a thick candy coating on a delicious gobstopper. Each layer is slightly bigger than the one before.
• Slices become wedges, identical sections like slices of an orange.
• Boards become plates, thick discs which can be stacked together. (I sometimes daydream of opening a bed & breakfast that only serves spherical stacks of pancakes. And they say math has no real-world uses!)

The 3d steps can be seen as the 2d versions, swept out in various ways. For example, spin the individual rings (like a coin) to create a shell. Imagine slices pushed through a changing mold to make wedges. Lastly, imagine we spin the boards to make plates, like carving a wooden sphere with a lathe (video).

The tradeoffs are similar to the 2d versions:

• Organic processes grow in layers (pearls in an oyster)
• Fair divisions require wedges (cutting an apple for friends)
• The robotic plate approach seems easy to manufacture

An orange is an interesting hybrid: from the outside, it appears to be made from shells, growing over time. And inside, it forms a symmetric internal structure -- a better way to distribute seeds, right? We could analyze it both ways.

Exploring The 3d Perspective

In the first lesson we had the vague notion the circle/sphere formulas were related:

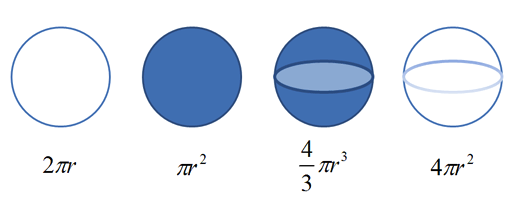
Well, now we have an idea how:

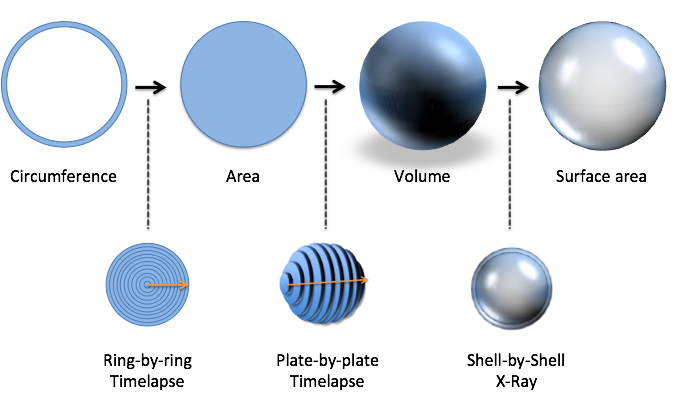
• Circumference: Start with a single ring
• Area: Make a filled-in disc with a ring-by-ring time lapse
• Volume: Make the circle into a plate, and do a plate-by-plate time lapse to build a sphere
• Surface area: X-Ray the sphere into a bunch of shells; the outer shell is the surface area

Wow! These descriptions are pretty detailed. We know, intuitively, how to morph shapes into alternate versions by thinking "time-lapse this" or "X-Ray that". We can move backwards, from a sphere back to circumference, or try different strategies: maybe we want to split the circle into boards, not rings.

The Need For Math Notation

You might have noticed it's getting harder to explain your ideas. We're reaching for physical analogies (rings, boards, wedges) to explain our plans: "Ok, take that circular area, and try to make some discs out of it. Yeah, like that. Now line them up into the shape of a sphere…".

I love diagrams and analogies, but should they be required to explain an idea? Probably not.

Take a look how numbers developed. At first, we used very literal symbols for counting: I, II, III, and so on. Eventually, we realized a symbol like V could take the place of IIII, and even better, that every digit could have its own symbol (we do keep our metaphorical history with the number 1).

This math abstraction helped in a few ways:

• It's shorter. Isn't "2 + 3 = 5" better than "two added to three is equal to five"? Fun fact: In 1557, Robert Recorde invented the equals sign, written with two parallel lines (=), because “noe 2 thynges, can be moare equalle”. (I agrye!)

• The rules do the work for us. With Roman numerals, we're essentially recreating numbers by hand (why should VIII take so much effort to write compared to I? Oh, because 8 is larger than 1? Not a good reason!). Decimals help us "do the work" of expressing numbers, and make them easy to manipulate. So far, we've been doing the work of calculus ourselves: cutting a circle into rings, realizing we can unroll them, looking up the equation for area and measuring the resulting triangle. Couldn't the rules help us here? You bet. We just need to figure them out.

• We generalized our thinking. "2 + 3 = 5" is really "twoness + threeness = fiveness". It sounds weird, but we have an abstract quantity (not people, or money, or cows… just "twoness") and we see how it's related to other quantities. The rules of arithmetic are abstract, and it's our job to apply them to a specific scenario.

Multiplication started as a way to count groups and measure rectangular area. But when you write "$15/hour × 2.5 hours = $37.50" you probably aren't thinking of "groups" of hours or getting the area of a "wage-hour" rectangle. You're just applying arithmetic to the concepts.

In the upcoming lessons we'll learn the official language to help us communicate our ideas and work out the rules ourselves. And once we've internalized the rules of calculus, we can explore patterns, whether they came from geometric shapes, business plans, or scientific theories.

Questions

1) What's your grandma-friendly version of the above?

2) Any aha moments or areas to clarify?