A sense of scale helps us better understand the world, and convey ideas more effectively. What’s more impressive?
- Bill Gates has 56 billion dollars.
- Bill Gates earned over \$3000 per minute (\$50/second) since Microsoft was created. Spending 5 seconds to pick \$100 off the floor is literally not a good use of his time.
If you’re like me, the second statement makes your jaw drop. 56 billion is just another large number, but \$3000 per minute is something vivid and “imaginable”. Let’s check out a few ways to convey a sense of scale.
Compare Side By Side By Side
A common way to put things in perspective is to literally line them up, side by side. We’re visual creatures. We like to see, not imagine abstract numbers. To our brains, a million, billion, and trillion all seem like large, vague numbers.
Apple knows this. Many of its ads compare products to everyday objects, rather than touting the raw dimensions:

The Macbook Air fits into a manilla envelope. The ipod nano is as thick as a pencil. Certain cameras fit in a box of altoids. You know their size without busting out a ruler. Just yesterday, I got a haircut with the #5 clippers (“As wide as your finger”) and knew what it meant. The hairdresser didn’t have to say “.875 inches”.
It seems backwards that “casual” measurements like a pencil’s width can be more useful than a count of millimeters. But we’re not machines — our everyday experience is with pencils, not millimeters, and we can easily imagine how much room a pencil takes.
Here’s a few more examples of side-by-side comparison in action — notice how well they convey a sense of scale.
Relative size of planets & stars. A great example, much preferred to “Boys and girls, the Sun’s diameter is 1000x larger than the Earth’s”.
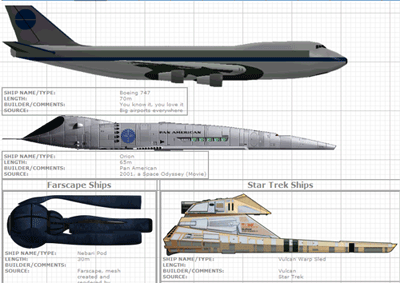
Relative Dimensions of Fictional Ships & Characters. Fun and interesting: occupy a geek for hours by asking how many TIE fighters would be needed to take out the Starship Enterprise.
Interactive Sense of Scale Flash App. A fantastic way to visualize the relative sizes of objects.
And of course, the famous power of ten video:
Rescale and Resize
Instead of looking up at the “big numbers”, we can shrink them to our level. Imagine the average person makes 50k/year, and a rich guy makes 500k/year. What’s the difference?
Well, instead of visualizing having 10x your money, imagine that things cost 10 times less. A new laptop? That’ll be 150 bucks. A new porsche? Only 6,000 dollars. A really nice house? 50k. Yowza. Things are cheap when you’re rich.
To understand Bill Gates’ scale, don’t think of 50 billion dollars and 5 billion/year income — it’s just another large number. Try to imagine having things cost 100,000 times less (and 100,000 is a pretty large number).
A laptop would be a few pennies. A porsche would be about 60 cents. Your \$50M mansion would be a mere 500 bucks. You could “splurge”, spend \$1000, and get everything you’ve ever needed. And you’re still earning 50k/year.
It’s much more vivid than “50 billion in the bank”, eh?
Use What We Know: Time and Distance
Sometimes, a different type of scale may be useful. We know time and distance, which cover a surprisingly broad range of sizes.
For most of us (myself included), millions, billions and trillions are “big”. It’s not intuitively obvious that a trillion is actually a million squared — that is, a trillion makes 1 million look imperceptible.
Check out these brain-bending figures:
- 1 second is 1 second
- 1 million seconds is 12 days (a vacation)
- 1 billion seconds is 30 years (a career)
- 1 trillion seconds is 30,000 years (longer than human civilization)
Yowza. Do you feel the staggering difference between a trillion and a million? Between a billion and a million?
We get a similiar effect when thinking about distance:
- 1 millimiter is 1 mm (pretty tiny)
- 1 million mm is a kilometer (down the street)
- 1 billion mm is a 1000 km (600 miles — partway across the country)
- 1 trillion mm is 1,000,000 km (Going around the world 25 times, almost as wide as the Sun)
Again, see the difference? How small a million is (“down the street”) compared to the size of the Sun?
These numbers come in handy in many applications:
- 99.999% reliability (“Five 9′s”) means an error rate of 10 out of a million. That is, you can be offline for only 10 seconds every 12 days. Or, you can have a tolerance of 10mm for every kilometer. That’s pretty accurate!
- “One part per million” is often used by chemists to measure concentrations of substances. One ppm is like having a presence of 1 second in 12 days. And a part per trillion? You got it: 1 second every 30,000 years. That’s tiny.
This approach helped me understand how utterly gigantic a trillion is, and how precise 99.999% really is.
Use People, Places and Things
Yet another approach is to combine things we’re familiar with. Here’s a few numbers:
- There’s about 6.5 billion people on Earth
- The internet has many billions of pages (call it a trillion to be safe)
The US deficit of 10 trillion dollars would require a tax of \$10 for every page on the internet to pay off (Yowza! And these are with generous estimates of the internet’s size).
A GUID, or large ID number used in programming, is at no risk of running out. How many are there? Well, we could give everyone a copy of the internet, every second, for a billion years… and still have enough GUIDs to identify each page. See how much bigger that is than “2^128″? (For the geeks: yes, the birthday paradox makes the chance of collision much higher).
Seeing a number impact the real world (i.e. being applied to every page of the internet) makes an idea come to life.
Summary
This article isn’t really about numbers. It’s about understanding and communication, how we think and convey ideas. Do you insist on rigid scientific terms, or do you reach out to your audience with terms they understand? Do you think a “lay person” (someone who happened to choose a different field of study than you) is more interested in raw numbers, or side-by-side demonstrations?
Developing a sense of scale helps us better understand the world and better convey that understanding.
In a perfect universe, we’d hear “one trillion”, imagine a million by million grid, and say “wow”. But that’s not the case — in order to say “Wow!” we need (or at least I need) to imagine the number of seconds in 30,000 years, longer than modern human civilization.
When presenting ideas, remember that analogies can be more powerful, interesting and effective than a 1 with 12 zeros.